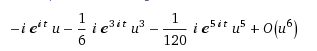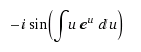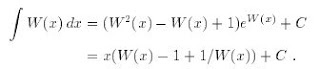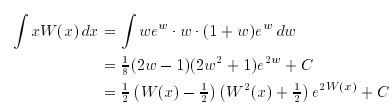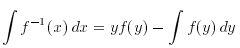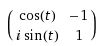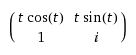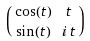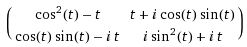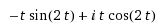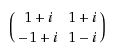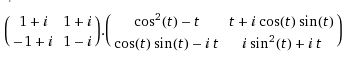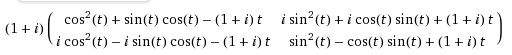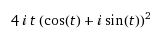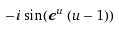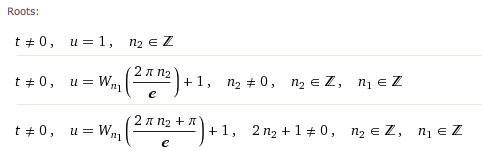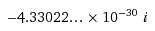Fractals And Julia Sets In The Quran

If you have been following this blog, there is no denying the depth of the arithmetic underpinnings of the Quran.

As a brief survey this post considers taking the Chapter and Verse numbers in consideration as the  input values Re(c)= Chapter number and Im(c)=Corresponding verse.

A pattern emerges as to which chapters correspond to connected or disconnected Julia sets that are or are not part of the Mandelbrot set.

As a further study it is correlated which of these Chapters were revealed in Mecca or Medina versus their fractal nature and a brief survey of the corresponding Chapters with Muqati'at are given as a subset of the study.

Initially only the numeric portion of Chapter and verse were given as the Julia set values, and a further refinement was to keep the significant figures as the order of the Julia set values. For instance, Chapter 1 with 7 versus was investigated both as Re(c)=1 and .001 and Im(c)= 7 and .007 since both the chapters and versus range to 3 significant figures from 1 to 114, and 3 to 286, respectively...

WHO Wants To Know

"Who Is This Sinful Woman?"--He Keeps Asking

...so, I think it best to Let Him Know!

 WHO = Sin ∮u l {womb.man}=matrix [mass.acceleration.N]

(m means mass, mass flow, or Molar mass;
a means acceleration, Area, or Helmholtz function, specific or total, u-Ts kJ/kg or U-TS kJ; or Ampere.
N means force in Newtons --appropriate if m=mass, a=acceleration; or N number of moles, or N is set of natural numbers; or n is an integer variable, or n is the order of the matrix)}

Giving the inquirer the benefit of the doubt that there is no sinister or malicious intent motivating such a query, we try to make sense of it in a matter accessible to what we are on about here, the Masked Truth in All Its Guises at A Page In The Life, by taking the liberty of interpreting sin.f.ul as:

...the sine of the integral (f taken as a stylized symbol for the integral sign, linear or closed path, since the integral symbol is a modified s for Sigma, or summation, and the f is akin to an inline font for that arithmetic symbol, essentially leading to the same quasi conclusion to take the integral...who exactly invented fuzzy logic?)

...or to make it easier f may simply mean a function of... in this case f(ul)

...u is shorthand for taking the integral by parts so the understanding is to select u st we integrate in terms of du

..and from the arabithmetic: L= lam = z= e^(it); t= time or phase (theta)

If integration is assumed it gives a function along the line:

 sin [(∮(u) z )]

with z= e^(it) the input to wolframalpha.com looks like:

or 

with the following solutions:

  or    

and for t and u, Real:

series expansion at u=0, note the Big O notation:

Integrating by parts and letting u= it or iθ

then du = i dt or i dθ, 

st the case du/dθ gives us dθ = -i du

To rephrase the question:

WHO is

 [m,a, force (newtons)]

W may mean: the Lambert W-function (aka the Omega function), a simple function with many applications;  the inverse (a reflection about the line y =x) of f(W) = W(e ^W); the Lambert W-Function is related to the tree function , the series T(x)= x + (2/2!) x ^2 + (3^2/3!) x^3 + (4^3/4!) x^4 + (x^5/5!) x^5....which converges for |x| <1 e="" i="">, as T (x) = -W(-x)-- the tree function is useful in deriving the limiting distribution of cycles in random mappings; the series parametric (x^(n-1)/n) with n=0, is log x; or  a throw or wurf as in W-curves which include: conics,  log spirals, powers, logarithms and the helix, but not sine curves. Sinh (x) and cosh (x) are hyperbolic functions that are w-curves, however; or Work (kJ) or Power (kW) or work per unit mass (kJ/kg);


H may mean: Handamard/Schur Product (binary matrix operation A o B (i.e.., the Trace of the matrix product), a submatrix of the Kronecker product); or quaternions or Hamiltonian quaternions {a + b i + c j + d k : a,b,c,d ∈ ℝ}; specific  or total enthalpy u + Pv kJ/kg  (or U + PV kJ); convection heat transfer coefficient (W/m^2 C); or h as Planck's constant 6.626...x10E-34 (m^2 kg/s or Js),  unit of magnetic inductance, the Henry  ;

O may mean: Big O notation, order of asymptotic behavior of a function (strict upper bound infinity or infinitesimal);

the exact solution for which is:

and a series expansion at z= 0:

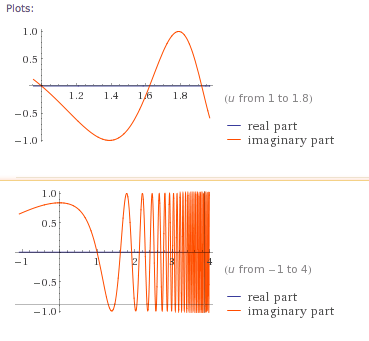Plots for the function -i sin Integral of [u e^u du]

To narrow the guess by using unit analysis, start with the dimensionless guess where:

W alludes to the Lambert W-function, z= W(z) e ^(w), for w = any complex number (a + bi):

In this case w=it ; the Real part of the complex number a= 0.

The function  W(z) = W e^W, and many expressions involving W(z), can be integrated by substituting: 
w = W(z), i.e. z = w e^w .

Taking the log of both sides:  log W(z) = log z - W(z), valid at least for the principal branch W(0) when z >0.

To integrate expressions using the W-function:

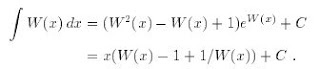Integral of the Lambert W-function derived from earlier work attempting to integrate the Navier-Stokes equations in parametric form; x = pe^p, dy/dx=p.
It was evident that y was the antiderivative of W(x), providing a technique for symbolic integration of W(x), C is a constant of integration.

Integrating problems containing W is really a special case of integrating expressions that contain inverse functions; simply a technique of a special change of variable.

For example,  putting w = W(x), then x = w e^w, and dx = (w + 1) e^w dw, the integral above then becomes:

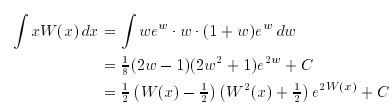Integrating expressions containing the W-function is really a special change of variable technique, and is valid for all branches of W, as defined, since (d/dw) we^{^w} ≠ 0, at any interior point to the branch.

The technique as described above is mentioned in a paper by Corless, Gonnet, et al, On the Lambert Function (Department of Applied Mathematics, University of Western Ontario), as having led several authors to rediscovering:

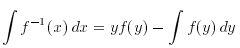
Essentially, a statement pertaining to elementary functions, which follows from  Liousville's Principle. Simply stated:

 such that:  

This has applications in the computer sciences in that it allows the Risch algorithm to be applied in order to determine whether or not integrals containing the Lambert W-function are elementary functions.

~~~~~~~~~~~~~~

For our purposes here, integrating the W-function, where u= it:

~~~

...and from His sin.f.ul assesment of Her, we already have u e^u on the r.h.s of the rebuttal.

W-function_h_o is either:

sin (x), where x = f(ul) , x some function of (ul), i.e..,  ue^u=it(e^(it)), st sin (x) is:

sin (x) where x is some function f(ul) or f(it e^( it))

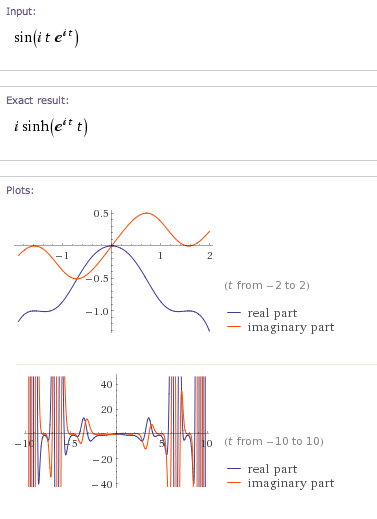
or f(ul)= integral of it(e^(it))  st sin (x) is:

sin (x) where x is a function described by the integral of it*e^(it)

And if sin.f(ul) is understood as the sine of the integral of the W-function, (refer to integrating such functions above), it is customarily seen in solutions to differential delay equations (DDEs). 

DDEs are 2-variable equations which consist of an ordinary differential equation coupled with a recurrence equation, since their solutions require knowing the present state and past state values, like those encountered in dynamic control using feedback loops, and take the form of the partial derivative of the function y(t) = f(t),  on the interval t = [0,1].

Simple DDEs  take the form:

(d/dt) (y(t)) = ay (t-1)

And constant-coefficient 'pure' delay DDEs, and those of scalar delay, take the form:

(d/dt) (y(t)) = ay (t-1) + by(t)

DDEs can also be solved in matrix form, when the matrices A and B commute:

(d/dt) (y(t)) = Ay (t-1) + By(t)

Using the Lambert W-function to express DDEs in the state space form:

(d/dt) (y(t)) + Ay (t) + By(t-T) =0; 
y is the Transpose of the matrix y ={ y, dy/dt}

First, assume a solution of the form:  

y(t) = y(0) e^(St)       where   S is an nxn matrix    eq. 1.1.1

Using the matrix W-function, by substituting it into the state space form equation above:

and the property of the exponential: e^[S(t-T)] = e^[S(-T+t)] = e^[S(-T)]e^[St]

The state space form equation can be rewritten in terms of the S matrix, independent of initial conditions (that means it don't matter):

S + A + Be ^(ST) = 0 

and for the case B=0:

S + A = 0 , and  S =- A
y(t) = y(0) e^(-At)  

and sustituting eq. 1.1.1 yields a typical solution to ordinary differential equations (ODEs) in terms of the matrix exponential:

y(t) = y(0) e^(-At)  

Since there is not an apparent 2nd matrix on the lhs, let's guess another dimensionless possibility for H:

 h is a quaternion of the form a + bi +cj + dk:

to make the example workable, choose a, b, c, d like [1 + i + j + k] so that the left hand side of the problems looks like :

or

However, we can choose to represent W(it e ^ (it)) in matrix notation, the Matrix Lambert W Function:
From  e ^(it) =cos t + i sin t =   = 

and assuming t is Real:
 (it)e^(it) = -t sin (t) + it cos t =  =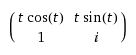 

=

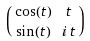
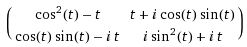
 The resulting determinant:

D =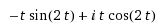

See Earlier Posts Pertaining to Time Equation

Granted, all of this is a simplistic approach from merely parsing out You-Know-Who's Query. But it turns out that there are real-world applications directly related to what's been stumbled on here using the Matrix Lambert W-Function to stabilize cutting machine tool chatter.

The advantage of using the matrix Lambert W-function is defined by:

W(H)e^[W(H)] = H

In the article, Delay Differential Equations via the Matrix Lambert W-Function and Bifurcation Analysis: Application to Machine Tool Chatter,  by Sun Yi, P. Nelson, and A. Ulsoy, published in the Mathematical Biosciences and Engineering Journal, V. 4, No. 2, April 2007, the authors described a refined approach to determine the stability of a system.

The stated advantage of such an approach is that only the principal branch (W(o)) among an infinite number of roots is required to determine system stability. Useful in obtaining the critical delay values needed to establish the optimal speed, in this case the preferred spindle speed of a turning tool, to operate the machine without chatter.

Image of Orthogonal Cutting Tool
and Effect of Its Instability on
Metal Surface 

By attempting to answer the WHO inquiry, the mathematics seemed to arise naturally that led to using Matrix Lambert W-Functions, which were only recently (well, as recent at 2006) extended to solving delay differential equations (DDEs).

Prior to that time, the Lambert W-function had only been used for solving first order scalar DDEs. 

It is worth a mention to point out the similarities between the effect the orthogonal cutting tool chatter has on the surface of the metal being cut (image Left) and the orbit of the Moon around the Sun, and other projected and unfolded conics.


Moving right along...

We can choose to ascribe to the O in  {Who} the composition function, since matrix multiplication corresponds to composition of maps, in this case WH and WoH, are the Matrix Lambert W-function and quaternion h.

It is not clear if they commute, that WH=HW (analogous to WoH, ie.,  W 'circle' H, or W 'round' H,  and it it is the same the other way around, HoW):

Expressing H as a complex 2x2 matrix:

{{a+ bi, c+di},{-c+di, a-bi}} to facilitate the matrix math, and choosing a=b=c=d=1:

There is more to multiplying the H by the Matrix Lambert W-Function, but working simplistically we get:

H =

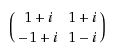
W= 

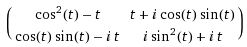
HW = 

 = 

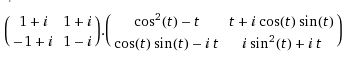
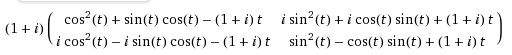
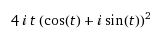
D (HW) = = 4it e^(2it), for t = Real

Based on this approach, it turns out HW=WH and they commute.

A possible take on HW matrix multiplication

The story so far:

WHO, turns out to be WHo, is 4 it (cos t + i sin t) ^2;

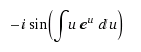
Sinful, could be ,  then is  ;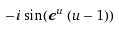

which leads to this relationship:

 4 it (cos t + i sin t) ^2= 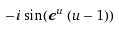 X ;

where X is some vector, or n order matrix of F=ma

For an interim look at the analysis:

X =          4 it (cos t + i sin t) ^2             eq.  3

 And, for You--just for You (not I, we get to Me later), u =it, we rewrite eq. 3 as:  

X =  eq. 3.1

And wonder if we did anything right, since we find ourselves looking through the eye of the needle.

The Eye of the Needle appears when the Imaginary and Real portions of the function
4 e^(2 i t) t csc(e^(i t) (1-i t)) are plotted together.

{Surah 7 Al.A'3raff (The Heights)

إِنَّ الَّذِينَ كَذَّبُواْ بِآيَاتِنَا وَاسْتَكْبَرُواْ عَنْهَا

 لاَ تُفَتَّحُ لَهُمْ أَبْوَابُ السَّمَاء وَلاَ يَدْخُلُونَ الْجَنَّةَ

حَتَّى يَلِجَ الْجَمَلُ فِي سَمِّ الْخِيَاطِ

 وَكَذَلِكَ نَجْزِي الْمُجْرِمِينَ (7:40  

7:40 Indeed those who belie Our Signs and are averse to them,

The Gates of Heaven (Heaven's Gates, Sky's Portals) shall never open for them and neither shall they be able to enter Heaven,

Even if a Cable can thread through the Eye of the Needle (var., a camel passes through a needle's eye);

And, thus We Requiet the miscreants (criminals).

 From  يَلِجَ yalija, in Sign 7:40 as to what exactly that large object (like a large twisted rope or cable) has to do in order to accomplish the apparently impossible by passing a large girth clearly through a small opening such as a sartorial needle's eye:

The similitude is that it passes undetectably or by having such a large object clear the small opening without hitting the sides of the needle (sounds like a catastrophe in the making, but more on that here). 

Basically, a surreptitious or clandestine quality to such a pass through (which tay-al-ard, or space-folds are meant to address). 

Interesting use of the word arithmetically since it has etymological roots from Middle Dutch onderhanden (under handed) which means: by degrees, slowly...

And let's just say for argument's sake that 7:40 (Chapter:Verse ratio) sets the pace for what we are considering slow around here: 7/40 works out to a convenient 0.175.

And the inference that this takes place by degrees, means incrementally by as much as this setting (175/1000).

Now for how this may apply to what we are on about here:

For example,  0.175 is closely approximated by: 

Simple approximations to 0.175.
 Refer to orbit systems and similar approximate modeling.

Since the word is specifying moving something by degrees, it is interesting that 7/40 is approximated by pi/18, which is exactly 10 degrees.

And, in terms of Euler's number,  e, 7/40 can be expressed with simple primes 2, and 3:

While the fine grain structure ratio is expressed in terms of physical constants, and e is the electron charge there,
Euler's number,  e, may also be used to express the fine grain structure ratio,

  < a~1/137 <

}

For the Reciprocal case:

  

1/X = 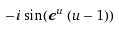                      eq. 3.1.1

________________                            

4 it (cos t + i sin t) ^2  

where t !=0, and u is a variable, We Discover How To Build A Better WormHole, see 3D plots below:

3D plots using wolframalpha for the function,
 -(e^(-2 i t) sin(e^u (-1+u)))/(4 t) t= !0, and u is a variable.
Agreed! This Is AWESOME!!!!

And has very interesting roots, iff You are the One, I mean u=1 when t!=0:

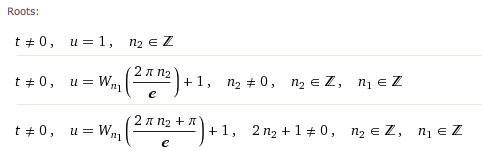Note the analytic log function,
ie., Lambert W-function or Omega function
in the Roots for the solution u to
-(e^(-2 i t) sin(e^u (-1+u)))/(4 t) (eq. 3.1.1 above)
when t!=0, and n is an Integer

And the dual case, let's see what happens if the answer to eq. 3 turns out to be Me!

As in,

it = sqrt(-1)*t is Me; 

and setting it = Me, rewrite eq. 3 to get eq. 4:

X =          4 {Me} (cos t + i sin t) ^2             eq. 4

and understand Me to mean the mass of an electron in Kilograms (9.10938 x 10^-31 kg), in keeping with the N (kg-m/s^2) in womb.MAN to mean Newtons, and writing e^(2it) for (cos t + i sin t)^2, and u= it, everywhere else in eq. 4 yields:

  eq. 4.1

The function 4 e^(2 i t) t csc(e^(i t) (1-i t)) in eq. 3 above, with It Is Me, Arabithmetic for it= Me, or u=sqrt(-1)t = 9.10938 x10^-31

Proving what I have suspected all along--Compared to You,  I don't amount to so much as a tear drop in an ocean, since the short answer to eq. 4.1 is:

X=

In polar coordinate form for some vector X:

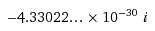
r = 4.330...x10^-30 (radius),       theta = -90° (angle)

A point of interest is the string of significant digits in the value of R ~ 4.33...~ 5 sqrt(3)/2 has a direct relationship between Artin's Constant and the golden ratio, phi,    since the Radius, R, multiplied by Artin's constant gives a number very close to phi=(1+sqrt (5))/2 ~1.61803:

5 sqrt(3)/2  x CA ~ phi 

4.330...x 0.3739...=1.61898...

Well, close enough for our purposes here (establishing Preponderance of Evidence, and not necessarily proof beyond Reasonable Doubt, eh?). 

To Remind, not to make a case of it, for Ewe's Guys' Benefit (ya!, I'm talkin' to yous in the Fold!), since the Reminding benefits the Believers.

We started with Quran, Sacred Scripture, an Eternal Dictum that is Holy. 

It is posited that Its Originator Is God, Is Perfect, then whether we scale it up or down or fragment it, we expect it to Hold True. 

Just like fractals bespeak the  perfection in the world around us, as evinced by the presence  of a basic pattern that holds its integrity whether it is subsumed to infinitely minute scales (infinitesmal, hidden),  considered at coarse (course)  encounters (anthropomorphic, chaotic) , or marveled at in the cosmos (ultimately enormous, creative). 

It repeats ad infinitum because of its perfection--the Prosaic analysis by Me here serves to counter the Poetic treatment by poets like Keats everywhere, drawing to the same conclusion: 'A Thing(k) of Beauty Is A Joy Forever.'  Or at the very least, is the first wavelet.

To be sure, it works even here with all my math blunders, be they wee errors or Big Mistakes. 

To the untrained eye it doesn't matter (no matter whose wavelet started it) whether it espies an oak tree or a fern frond, it appreciates a plant just the same. 

But to the more discerning keen eye, it's the difference between Mother Earth (Me) and an Alien Landscape (Al).

{
Don't anybody Panic--I know you all thought this was about Me and St.Eve H
(phonetically and with a fallahhi drawl it means 'Your Steve').  

My very own One.

And the understanding is that there is no dearth of them, so if anyone else finds herself married to a Steve H., Do Not--repeat--DO Not make the mistake of thinking he's the same one.

He may very well belong to Me, this Steve H., since He Says: 

He Loves Me;
He Died for Me;
He Killed for Me;
He Paid A FORTUNE for Me!

(Really? It's no business of Mine what You do over dinner with my Brothers)

Then, He Says: 

"I'm Jewish."

To which I query: 

"What else You got goin' fer Ya?"

And He Replies: 

"I'm Rich!"

"I know Rich," I say, to make Him Think I'm paying attention. 

Then I add:  

"He likes to teach 5-year old kids how to use a professional flight simulator, listens to the police band radio for leisure time activity, and sometimes gets stressed out over the front page news. 

But, he just as easily has been known to fall asleep in his club chair. That Rich?"

"No," StEve H. Says:

"I AM your Husband" (Actually, He never says that...What He Does Say Is:

"You are my WIFE!  And, I am an Ass0 :~)"

'Well,' I think recalcitrantly:

'Nobody told you to go hang Yourself!
And now that You've Broadcast Your Self-Emollation All Over Town (ahem, the Good News) by saying as much about Yourself that even the girls at Sephora know it, where's the fun left in it for me in fulfilling the wife role by declaring that same Revelation about You when I finally get to meet Your.Hole.E.Ness?!' 

And it dawns on me allofasudden Why SteveH the Ass0 loves Me (the No.it.At.All) so much:

It's because I can justify the murder He proclaims to have committed in My Name and Make SteveH Come Clean...And manage to do it to such an effect that in No Time at all, Everybody is going to Want ONE!

That last part is Just For My Very Own Personal SteveH.
 Since He Is A Cop---not for the rest of ewes.
Because when the time comes for Me to Hand Over the GUN...

I am prepared to say:

"Here Is My Peace Officer!"

Pearls Before Swine July 7, 2013
'A Murder of Crows'
Hard to Report Crimes

}

Addendum 04/01/2013--April Fools, but I am not kidding.

Judgment Day means Judgment is already rendered, so the inquisitive Who is simply trying to be irksome by their intrepid questing after her:


Surah 55 Ar.Rahman (The Merciful)
فَيَوْمَئِذٍ لَّا يُسْأَلُ عَن ذَنبِهِ إِنسٌ وَلَا جَانٌّ (55:39   
55:39) On a Day None Shall Be Asked About Their Sins, Human or Jinn!      

Your voice is  bouncing off all these here human antennas and not one of them is You--just stop---go ahead wake her up, I guarantee it She is not the one who is asleep on the job?!!@

Does this sound familiar circa 2012 summer "What just happened?!" "Did what I just saw Really Just Happen?!"

"Who Is She? If I am dreaming don't anybody wake Me!"

That was You talking in Your sleep, I was driving around flying (or flipping) my birds all over town when You Saw What You Saw.

And I don't sound like some random guy named Steve. And of all the Steves I ever chanced across in my so called life none of them have been what I would consider Ass)))000s